Talk:Rule of Sarrus

Error in formula

someone should check the sarrus formula, i think there is an error the third term is a13 a21 a32 and not a13 a21 a23 —Preceding unsigned comment added by 194.138.12.144 (talk) 13:16, 26 February 2008 (UTC)[reply]

4x4 matrix?

Will this work the same for any sized square matrix? or is it limited to just 2x2 and 3x3? —Preceding unsigned comment added by 68.95.143.218 (talk) 12:51, 10 September 2008 (UTC)[reply]

No, it won't (as mentioned in the article). This simple scheme only works for 2x2 and 3x3 matrices.--Kmhkmh (talk) 15:59, 27 January 2009 (UTC)[reply]

Please prove this

it's in the sources - i.e. read them--Kmhkmh (talk) 14:03, 24 June 2009 (UTC)[reply]

pictures/illustrations

There is no point in (arbitrarily?) adding additional illustrations for Sarrus' rule. Commons have quite a number of them by now and it make sense to provide a commons link, but for the article one of them is sufficient. Furthermore the newly added redundant picture messes up the display in many browsers as the math formula and the picture overlap creating a large empty gap and the picture does illustrate the exact text of the article either (displaying a row based version rather than the column based version).--Kmhkmh (talk) 18:42, 4 May 2014 (UTC)[reply]

The picture you removed has some additional value over the present one (showing pluses and minuses at least) and I am not trying to speak about information diversity here, which too is hurt by removing a relevant quality content. So you removed an image with an added value, which is bad. The reasons you state are not relevant to the image, but to a possible error in text parsing. You should file a bug to Bugzilla trying to resolve that instead. I will not revert the change again but please try to think about my reasoning first.--Kozuch (talk) 20:41, 4 May 2014 (UTC)[reply]

Sorry, I don't agree on the additional value, it it just a slightly different representation. And whether the plus and minus is in the graphic itself or in the subtext attached to the graphic makes little difference. As far as the reason I've stated, they are not relevant to the image itself, but they are relevant to overall article, its content and the placing of additional images in it. As far as the display problem is concerned, that is not a bug, but a wikipedia/html property if you have several (too many) pictures competing for space. The solution to that is to reduce the number of images to the ones you really need (note that math formulas/latex parts get transformed into images and you have the main math formula and your image competing for space).--Kmhkmh (talk) 23:51, 4 May 2014 (UTC)[reply]

recent edit

^{This discussion is related to this edit: 889295280}

I see no real benefit in the recent edit. First of all it now suggest the 2x2 is also called rule of Sarrus, which to my knowledge (and the sources used for the article) is wrong, as only the 3x3 case is called Sarrus rule in them.

That factual error aside which purpose does it serve to blow up the number of sections without actually adding content? This is a rather small article (smaller than the lead of misized article), where adding additional sections is neither necessary nor really improving navigation but imho just interreupting the flow of reading.

Furthermore the see also section is neither needed nor recommended here either as the Laplace extension is already mentioned and linked in the article main body (see MOS:NOTSEEALSO)--Kmhkmh (talk) 19:38, 24 March 2019 (UTC)[reply]

@Kmhkmh: Well the primary reason of why I've made changes in the first place is that intro doesn't explicitly states that method is applicable to only 2x2, 3x3 matrices. So it was simply clarified.

[...] First of all it now suggest the 2x2 is also called rule of Sarrus, which to my knowledge (and the sources used for the article) is wrong Even though I haven't changed (questioned) anything concerning correctness of the Rule applied to 2x2 matrices it is clearly works well for them. I can't imagine sources stating otherwise.

[...] sections is neither necessary nor really improving navigation but imho just interreupting the flow of reading. Sectioning is needed cause there are two subjects mixed together and it eases reading. I've been confused by the article as well: application of the rule for 2x2 is a little bit different from that for 3x3 and it was unexpected to see it right there so I think must be kept separate and in proper order: 2x2 first, then 3x3. And sectioned of course.

Laplace extension is already mentioned and linked in the article main body Well I have to agree that manual can be applied here but it's clearly faster to find related article by links in the see also section rather digging up such a messed up article. I also think it would be better to move description of the origin into the the intro. Currently it's nearly unnoticeable. DAVRONOVA.A. ✉ ⚑ 22:19, 24 March 2019 (UTC)[reply]

Well I understand your (well meant) intention, I just saw/see it as a missinterpretation of the lead and hence the attempted fix as an unnecessary fix of a non-existing problem.

As far as the 3x3 and 2x2 cases are concerned. Only the 3x3 case is called Sarrus rule (as it was stated in the original lead). However adding the 2x2 case as a similar scheme to Sarrus' rule makes imho sense and some sources do exactly that. Note the sources only call the scheme for 3x3 matrices Sarrus rule, but some mention a similar scheme for 2x2 and point out that for >3x3 a similar scheme does not exist.--Kmhkmh (talk) 22:51, 24 March 2019 (UTC)[reply]

@Kmhkmh: Only the 3x3 case is called Sarrus rule (as it was stated in the original lead) The intro doesn't restrict rule explicitly to other matrices. You can't simply ignore it by calling it as non-existing problem. Regarding how often people tend (even in this section) to extend the rule to larger matrices the only way to address the problem is to explicitly state that it is not applicable. I proposed the way to do that: move statement on the origin (which is about Laplace expansion) into the intro. Do you agree or not?

However adding the 2x2 case as a similar scheme to Sarrus' rule makes imho sense and some sources [...] I question only the order in which both cases are described. The 2x2 should be either placed before or after 3x3 as a notion. Agreed? I also would like to separate both cases by section as I did before and ain't agree on anything else unless mess with two subjects is cleaned.

Note the sources only call the scheme for 3x3 matrices Sarrus rule [...] Would be nice to cite them using {{cite}} template. DAVRONOVA.A. ✉ ⚑ 09:46, 25 March 2019 (UTC)[reply]

@Kmhkmh: DAVRONOVA.A. ✉ ⚑ 09:10, 28 March 2019 (UTC)[reply]

Regarding the first question. I'm not particularly aware of people trying to apply Sarrus' rule to a 4x4 matrices. Whether you look it up here or in various sources it clearly states it is scheme for 3x3 matrices and nothing else. None of the sources I've looked at starts the description with pointing that Sarrus doesn't apply to 4x4 matrices. Also the lead of an article usually starts with its definition and not with its derivation/orig/proof. First an article defines its topic and only after that it can go on to describes how it relates to other topics or how it might be derived (like Laplace or leibniz). So no, I don't agree with your suggestion to move Laplace to the lead.

I also don't agreed with your suggestion to treat the 2x2 case first. As I've pointed out the 2x2 case is not called Sarrus rule, it is just something that looks slightly similar and can be memorized in a similar fashion. Maybe there is general misunderstanding here. This article is about Sarrus' rule and not about something like methods to compute the determinant of squared matrics. In the latter case I'd agree it would make sense to start with 2x2 and deal with that first, however this article is not the latter but the former.

Regarding the last question, I assume with cite you mean to footnote for that particular statement. Yes, we could do that and depending on your personal taste it might be seen as "slight" improvement. However it isn't really needed either, as the information is given in the sources at the end anyhow. As long as the article is this short and barely more than a normal paragraph it makes little difference whether you put the source in a footnote to a paragraph or simply list it at the end. You only really need footnotes when the article contains a lot of different statements from different sources and/or is changing a lot and/or has controversial/contentious content. This article however has been rather stable over the last 12 years of it's existence (see [1]) and has a rather noncontentious topic (or so I've thought at least).--Kmhkmh (talk) 02:41, 29 March 2019 (UTC)[reply]

@Kmhkmh: Thanks for expanded answer. Though you haven't answered my reasoning over sectioning. Does that mean that you agree to return some of them back?

Whether you look it up here or in various sources it clearly states it is scheme for 3x3 matrices and nothing else. Well considering that you've cited source (line 20) for the paragraph on the application of Rule for the 2x2 matrices your statement above only reinforces ambiguity. I haven't seen that book by myself, there is no quote either, so I would like to ask you to clarify how it come that the Rule that works for 2x2 is mentioned in the article meanwhile the scheme (I assume that by this word you meant the Sarrus rule) is only for 3x3 and nothing else. Anticipating your answer I would say that that's okay that both cases are described but I just would like to see them under a separate headings.

I think we can abandon discussion over application to a larger matrices.

This article is about Sarrus' rule and not about some like methods to compute the determinant of squared matrics. Why 2x2 case is listed then? Clearly, there is no other method for 2x2 matrices.

Also the lead of an article usually starts with its definition and not with its derivation/orig/proof. The intro of the article must contain summary on and overview of the article itself. You also may repeat facts already stated in the article (checkout the WP:SS style guide), even if the article is small. That's the purpose of the intro. I don't get how it would worsen article if you briefly state from where the Rule was derived.

Yes, we could do that and depending on your personal taste it might be seen as "slight" improvement. The every statement must be properly cited according to Wikipedia policy in order to make them verifiable.

article however has been rather stable over the last 12 years of it's existence Doesn't matter. DAVRONOVA.A. ✉ ⚑ 13:11, 3 April 2019 (UTC)[reply]

I thought was rather clear above. No, I don't agree with handling the 2x2 case first and no, I do not agree with your suggested structure for the reasons stated above. Basically that your structure misrepresents the sources and insinuates falsely the 2x2 would be considered Sarrus' rule. As I said above your structure would be appropriate if the article's topic was slightly different along the lines of "computation schemes or methods for determinants of small matrices" as title. You are free to create such an article, this article however is about Sarrus' rule.

As far as the lead is concerned, yes the lead is the summary and the smallest summary or minimal part of the summary is defining the article's topic. Also this requires a bit of common sense. If the article is already rather short, the "summary" or "lead" is usually just a lead sentence or introductory sentence. Note, there is little point in writing a more extensive summary of an article, the size of which is already smaller then most summaries.

As far as citing is concerned you are misreading the policy. There is no such policy requiring every statement to be cited, instead policy requires every statement to be verifiable. The latter is usually achieved by having a citation in the neighbourhood of that statement, which can word, sentence or paragraph based and a citation can source one or several statements. And as already mentioned above, in very short article providing the source at the end is essentially the same as citing paragraph based.

"Obviously" correct things don't need to explicitly sourced at all but just be verifiable. This matters in particular for math articles, so for instance in an article on most math topics you usually do not provide a citation on basic arithmetic just because the article contains a basic computation, unless the article is on arithmetic/basic computation itself.--Kmhkmh (talk) 15:21, 3 April 2019 (UTC)[reply]

First image

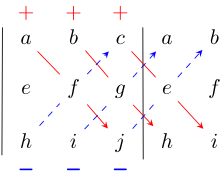
Is there a reason why the first image skips the letter "d" and goes from "abc" to "efg"? At first glance I found it a little confusing given that the written part of the article uses "abc" and then "d" – I had expected the image to simply illustrate what the text was saying. Perhaps the image ought to be adapted? AlexGallon (talk) 16:19, 3 June 2024 (UTC)[reply]