User:Mechatronicdip.gallecot

Mechatronic Diploma (Technical Collage - COT GALLE) NVQ Level 5

Mechatronics is a multidisciplinary field of engineering that includes a combination of systems engineering, mechanical engineering, electrical engineering, telecommunications engineering, control engineering and computer engineering.[1][2] As technology advances the subfields of engineering multiply and adapt. Mechatronics' aim is a design process that unifies these subfields. Originally, mechatronics just included the combination of mechanics and electronics, hence the word is a combination of mechanics and electronics; however, as technical systems have become more and more complex the word has been broadened to include more technical areas.

The word "mechatronics" originated in Japanese-English and was created by Tetsuro Mori, an engineer of Yaskawa Electric Corporation. The word "mechatronics" was registered as trademark by the company in Japan with the registration number of "46-32714" in 1971. However, afterward the company released the right of using the word to public, and the word "mechatronics" spread to the rest of the world. Nowadays, the word is translated in each language and the word is considered as an essential term for industry.

Description

Aerial Euler diagram from RPI's website describes the various fields that make up Mechatronics

A mechatronics engineer unites the principles of mechanics, electronics, and computing to generate a simpler, more economical and reliable system. The term "mechatronics" was coined by Tetsuro Mori, the senior engineer of the Japanese company Yaskawa in 1969. An industrial robot is a prime example of a mechatronics system; it includes aspects of electronics, mechanics, and computing to do its day-to-day jobs.

Engineering cybernetics deals with the question of control engineering of mechatronic systems. It is used to control or regulate such a system (see control theory). Through collaboration, the mechatronic modules perform the production goals and inherit flexible and agile manufacturing properties in the production scheme. Modern production equipment consists of mechatronic modules that are integrated according to a control architecture. The most known architectures involve hierarchy, polyarchy, heterarchy, and hybrid. The methods for achieving a technical effect are described by control algorithms, which might or might not utilize formal methods in their design. Hybrid systems important to mechatronics include production systems, synergy drives, planetary exploration rovers, automotive subsystems such as anti-lock braking systems and spin-assist, and everyday equipment such as autofocus cameras, video, hard disks, and CD players.

1st Semester (6 Months)

Engineering Drawing

• Objective:

To equip students with basic skills required in engineering drawings, electrical circuit

diagrams, and communication, Course Purpose and forms of communication; technical communication, report writing, drawing,

• Contents:

reprographics, engineering drawing, sketching, pictorial projections, paper sizes, scales,

conventions in layout, lettering and representation of components, tolerances, assembly drawings, K-parts list, exercises in machine drawings, structural drawings and design. Selection of machine components such as; V-belts, flat-belts and pulleys. Simple stress calculations for shafts and structural members. Course The Course Assessment (culminating to the Final Grade), will be made up of the

• Assessment:

following four components;

(i) Weekly Practical Reports
(ii) Practical Tests
(iii) Theory Tests
(iv) End of Semester Examination

Relationship to artistic drawing

Engineering drawing and artistic drawing are both types of drawing, and either may be called simply "drawing" when the context is implicit. Engineering drawing shares some traits with artistic drawing in that both create pictures. But whereas the purpose of artistic drawing is to convey emotion or artistic sensitivity in some way (subjective impressions), the purpose of engineering drawing is to convey information (objective facts). One of the corollaries that follows from this fact is that, whereas anyone can appreciate artistic drawing (even if each viewer has his own unique appreciation), engineering drawing requires some training to understand (like any language); but there is also a high degree of objective commonality in the interpretation (also like other languages). In fact, engineering drawing has evolved into a language that is more precise and unambiguous than natural languages; in this sense it is closer to a programming language in its communication ability. Engineering drawing uses an extensive set of conventions to convey information very precisely, with very little ambiguity.

Relationship to other technical drawing types

The process of producing engineering drawings, and the skill of producing those, is often referred to as technical drawing or drafting (also spelled draughting), although technical drawings are also required for disciplines that would not ordinarily be thought of as parts of engineering (such as architecture, landscaping, cabinet making, road construction and garment-making).

Persons employed in the trade of producing engineering drawings were called draftsmen (or draughtsmen) in the past. Although these terms are still in use, the non-gender-specific terms draftsperson and drafter are now more common.

Media

For centuries, until the post-World War II era, all engineering drawing was done manually by using pencil and pen on paper or other substrate (e.g., vellum, mylar). Since the advent of computer-aided design (CAD), engineering drawing has been done more and more in the electronic medium with each passing decade. Today most engineering drawing is done with CAD, but pencil and paper have not disappeared.

Some of the tools of manual drafting include pencils, pens and their ink, straightedges, T-squares, French curves, triangles, rulers, protractors, dividers, compasses, scales, erasers, and tacks or push pins. (Slide rules used to number among the supplies, too, but nowadays even manual drafting, when it occurs, benefits from a pocket calculator or its onscreen equivalent.) And of course the tools also include drawing boards (drafting boards) or tables. The English idiom "to go back to the drawing board", which is a figurative phrase meaning to rethink something altogether, was inspired by the literal act of discovering design errors during production and returning to a drawing board to revise the engineering drawing. Drafting machines are devices that aid manual drafting by combining drawing boards, straightedges, pantographs, and other tools into one integrated drawing environment. CAD provides their virtual equivalents.

Producing drawings usually involves creating an original that is then reproduced, generating multiple copies to be distributed to the shop floor, vendors, company archives, and so on. The classic reproduction methods involved blue and white appearances (whether white-on-blue or blue-on-white), which is why engineering drawings were long called, and even today are still often called, "blueprints" or "bluelines", even though those terms are anachronistic from a literal perspective, since most copies of engineering drawings today are made by more modern methods (often inkjet or laser printing) that yield black or multicolour lines on white paper. The more generic term "print" is now in common usage in the U.S. to mean any paper copy of an engineering drawing. In the case of CAD drawings, the original is the CAD file, and the printouts of that file are the "prints".

Engineering drawings: common features

Drawings convey the following critical information:

• Geometry – the shape of the object; represented as views; how the object will look when it is viewed from various angles, such as front, top, side, etc.
• Dimensions – the size of the object is captured in accepted units.
• Tolerances – the allowable variations for each dimension.
• Material – represents what the item is made of.
• Finish – specifies the surface quality of the item, functional or cosmetic. For example, a mass-marketed product usually requires a much higher surface quality than, say, a component that goes inside industrial machinery.

Line styles and types

Standard engineering drawing line types

A variety of line styles graphically represent physical objects. Types of lines include the following:

• visible – are continuous lines used to depict edges directly visible from a particular angle.
• hidden – are short-dashed lines that may be used to represent edges that are not directly visible.
• center – are alternately long- and short-dashed lines that may be used to represent the axes of circular features.
• cutting plane – are thin, medium-dashed lines, or thick alternately long- and double short-dashed that may be used to define sections for section views.
• section – are thin lines in a pattern (pattern determined by the material being "cut" or "sectioned") used to indicate surfaces in section views resulting from "cutting." Section lines are commonly referred to as "cross-hatching."
• phantom - (not shown) are alternately long- and double short-dashed thin lines used to represent a feature or component that is not part of the specified part or assembly. E.g. billet ends that may be used for testing, or the machined product that is the focus of a tooling drawing.

Lines can also be classified by a letter classification in which each line is given a letter.

• Type A lines show the outline of the feature of an object. They are the thickest lines on a drawing and done with a pencil softer than HB.
• Type B lines are dimension lines and are used for dimensioning, projecting, extending, or leaders. A harder pencil should be used, such as a 2H.
• Type C lines are used for breaks when the whole object is not shown. These are freehand drawn and only for short breaks. 2H pencil
• Type D lines are similar to Type C, except these are zigzagged and only for longer breaks. 2H pencil
• Type E lines indicate hidden outlines of internal features of an object. These are dotted lines. 2H pencil
• Type F lines are Type F[typo] lines, except these are used for drawings in electrotechnology. 2H pencil
• Type G lines are used for centre lines. These are dotted lines, but a long line of 10–20 mm, then a gap, then a small line of 2 mm. 2H pencil
• Type H lines are the same as Type G, except that every second long line is thicker. These indicate the cutting plane of an object. 2H pencil
• Type K lines indicate the alternate positions of an object and the line taken by that object. These are drawn with a long line of 10–20 mm, then a small gap, then a small line of 2 mm, then a gap, then another small line. 2H pencil.

Multiple views and projections

Image of a part represented in First Angle Projection

Symbols used to define whether a projection is either First Angle (left) or Third Angle (right).

Isometric view of the object shown in the engineering drawing below.

Main article: Graphical projection

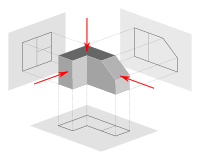
In most cases, a single view is not sufficient to show all necessary features, and several views are used. Types of views include the following:

Orthographic projection

The orthographic projection shows the object as it looks from the front, right, left, top, bottom, or back, and are typically positioned relative to each other according to the rules of either first-angle or third-angle projection. The origin and vector direction of the projectors (also called projection lines) differs, as explained below.

• In first-angle projection, the projectors originate as if radiated from a viewer's eyeballs and shoot through the 3D object to project a 2D image onto the plane behind it. The 3D object is projected into 2D "paper" space as if you were looking at a radiograph of the object: the top view is under the front view, the right view is at the left of the front view. First-angle projection is the ISO standard and is primarily used in Europe.
• In third-angle projection, the projectors originate as if radiated from the 3D object itself and shoot away from the 3D object to project a 2D image onto the plane in front of it. The views of the 3D object are like the panels of a box that envelopes the object, and the panels pivot as they open up flat into the plane of the drawing. Thus the left view is placed on the left and the top view on the top; and the features closest to the front of the 3D object will appear closest to the front view in the drawing. Third-angle projection is primarily used in the United States and Canada, where it is the default projection system according to ASME standard ASME Y14.3M.

Until the late 19th century, first-angle projection was the norm in North America as well as Europe; but circa the 1890s, the meme of third-angle projection spread throughout the North American engineering and manufacturing communities to the point of becoming a widely followed convention, and it was an ASA standard by the 1950s. Circa World War I, British practice was frequently mixing the use of both projection methods.

As shown above, the determination of what surface constitutes the front, back, top, and bottom varies depending on the projection method used.

Not all views are necessarily used. Generally only as many views are used as are necessary to convey all needed information clearly and economically. The front, top, and right-side views are commonly considered the core group of views included by default, but any combination of views may be used depending on the needs of the particular design. In addition to the 6 principal views (front, back, top, bottom, right side, left side), any auxiliary views or sections may be included as serve the purposes of part definition and its communication. View lines or section lines (lines with arrows marked "A-A", "B-B", etc.) define the direction and location of viewing or sectioning. Sometimes a note tells the reader in which zone(s) of the drawing to find the view or section.

Auxiliary projection

An auxiliary view is an orthographic view that is projected into any plane other than one of the six principal views. These views are typically used when an object contains some sort of inclined plane. Using the auxiliary view allows for that inclined plane (and any other significant features) to be projected in their true size and shape. The true size and shape of any feature in an engineering drawing can only be known when the Line of Sight (LOS) is perpendicular to the plane being referenced. It is shown like a three-dimensional object.

Isometric projection

The isometric projection shows the object from angles in which the scales along each axis of the object are equal. Isometric projection corresponds to rotation of the object by ± 45° about the vertical axis, followed by rotation of approximately ± 35.264° [= arcsin(tan(30°))] about the horizontal axis starting from an orthographic projection view. "Isometric" comes from the Greek for "same measure". One of the things that makes isometric drawings so attractive is the ease with which 60 degree angles can be constructed with only a compass and straightedge.

Isometric projection is a type of axonometric projection. The other two types of axonometric projection are:

• Dimetric projection
• Trimetric projection

Oblique projection

An oblique projection is a simple type of graphical projection used for producing pictorial, two-dimensional images of three-dimensional objects:

• it projects an image by intersecting parallel rays (projectors)
• from the three-dimensional source object with the drawing surface (projection plan).

In both oblique projection and orthographic projection, parallel lines of the source object produce parallel lines in the projected image.

Perspective

Perspective is an approximate representation on a flat surface, of an image as it is perceived by the eye. The two most characteristic features of perspective are that objects are drawn:

• Smaller as their distance from the observer increases
• Foreshortened: the size of an object's dimensions along the line of sight are relatively shorter than dimensions across the line of sight.

Section Views

Projected views (either Auxiliary or Orthographic) which show a cross section of the source object along the specified cut plane. These views are commonly used to show internal features with more clarity than may be available using regular projections or hidden lines. In assembly drawings, hardware components (e.g. nuts, screws, washers) are typically not sectioned.

Scale

Main articles: Architect's scale, Engineer's scale, and Metric scale

Plans are usually "scale drawings", meaning that the plans are drawn at specific ratio relative to the actual size of the place or object. Various scales may be used for different drawings in a set. For example, a floor plan may be drawn at 1:50 (1:48 or 1/4"=1'-0") whereas a detailed view may be drawn at 1:25 (1:24 or 1/2"=1'-0"). Site plans are often drawn at 1:200 or 1:100.

Scale is a nuanced subject in the use of engineering drawings. On one hand, it is a general principle of engineering drawings that they are projected using standardized, mathematically certain projection methods and rules. Thus, great effort is put into having an engineering drawing accurately depict size, shape, form, aspect ratios between features, and so on. And yet, on the other hand, there is another general principle of engineering drawing that nearly diametrically opposes all this effort and intent—that is, the principle that users are not to scale the drawing to infer a dimension not labeled. This stern admonition is often repeated on drawings, via a boilerplate note in the title block telling the user, "DO NOT SCALE DRAWING."

The explanation for why these two nearly opposite principles can coexist is as follows. The first principle—that drawings will be made so carefully and accurately—serves the prime goal of why engineering drawing even exists, which is successfully communicating part definition and acceptance criteria—including "what the part should look like if you've made it correctly." The service of this goal is what creates a drawing that one even could scale and get an accurate dimension thereby. And thus the great temptation to do so, when a dimension is wanted but was not labeled. The second principle—that even though scaling the drawing will usually work, one should nevertheless never do it—serves several goals, such as enforcing total clarity regarding who has authority to discern design intent, and preventing erroneous scaling of a drawing that was never drawn to scale to begin with (which is typically labeled "drawing not to scale" or "scale: NTS"). When a user is forbidden from scaling the drawing, s/he must turn instead to the engineer (for the answers that the scaling would seek), and s/he will never erroneously scale something that is inherently unable to be accurately scaled.

But in some ways, the advent of the CAD and MBD era challenges these assumptions that were formed many decades ago. When part definition is defined mathematically via a solid model, the assertion that one cannot interrogate the model—the direct analog of "scaling the drawing"—becomes ridiculous; because when part definition is defined this way, it is not possible for a drawing or model to be "not to scale". A 2D pencil drawing can be inaccurately foreshortened and skewed (and thus not to scale), yet still be a completely valid part definition as long as the labeled dimensions are the only dimensions used, and no scaling of the drawing by the user occurs. This is because what the drawing and labels convey is in reality a symbol of what is wanted, rather than a true replica of it. (For example, a sketch of a hole that is clearly not round still accurately defines the part as having a true round hole, as long as the label says "10mm DIA", because the "DIA" implicitly but objectively tells the user that the skewed drawn circle is a symbol representing a perfect circle.) But if a mathematical model—essentially, a vector graphic—is declared to be the official definition of the part, then any amount of "scaling the drawing" can make sense; there may still be an error in the model, in the sense that what was intended is not depicted (modeled); but there can be no error of the "not to scale" type—because the mathematical vectors and curves are replicas, not symbols, of the part features.

Even in dealing with 2D drawings, the manufacturing world has changed since the days when people paid attention to the scale ratio claimed on the print, or counted on its accuracy. In the past, prints were plotted on a plotter to exact scale ratios, and the user could know that a line on the drawing 15mm long corresponded to a 30mm part dimension because the drawing said "1:2" in the "scale" box of the title block. Today, in the era of ubiquitous desktop printing, where original drawings or scaled prints are often scanned on a scanner and saved as a PDF file, which is then printed at any percent magnification that the user deems handy (such as "fit to paper size"), users have pretty much given up caring what scale ratio is claimed in the "scale" box of the title block. Which, under the rule of "do not scale drawing", never really did that much for them anyway.

Showing dimensions

The required sizes of features are conveyed through use of dimensions. Distances may be indicated with either of two standardized forms of dimension: linear and ordinate.

• With linear dimensions, two parallel lines, called "extension lines," spaced at the distance between two features, are shown at each of the features. A line perpendicular to the extension lines, called a "dimension line," with arrows at its endpoints, is shown between, and terminating at, the extension lines. The distance is indicated numerically at the midpoint of the dimension line, either adjacent to it, or in a gap provided for it.
• With ordinate dimensions, one horizontal and one vertical extension line establish an origin for the entire view. The origin is identified with zeroes placed at the ends of these extension lines. Distances along the x- and y-axes to other features are specified using other extension lines, with the distances indicated numerically at their ends.

Sizes of circular features are indicated using either diametral or radial dimensions. Radial dimensions use an "R" followed by the value for the radius; Diametral dimensions use a circle with forward-leaning diagonal line through it, called the diameter symbol, followed by the value for the diameter. A radially-aligned line with arrowhead pointing to the circular feature, called a leader, is used in conjunction with both diametral and radial dimensions. All types of dimensions are typically composed of two parts: the nominal value, which is the "ideal" size of the feature, and the tolerance, which specifies the amount that the value may vary above and below the nominal.

• Geometric dimensioning and tolerancing is a method of specifying the functional geometry of an object.

Sizes of drawings

Main article: Paper size

ISO paper sizes

ANSI paper sizes

Sizes of drawings typically comply with either of two different standards, ISO (World Standard) or ANSI/ASME Y14.1 (American).

The metric drawing sizes correspond to international paper sizes. These developed further refinements in the second half of the twentieth century, when photocopying became cheap. Engineering drawings could be readily doubled (or halved) in size and put on the next larger (or, respectively, smaller) size of paper with no waste of space. And the metric technical pens were chosen in sizes so that one could add detail or drafting changes with a pen width changing by approximately a factor of the square root of 2. A full set of pens would have the following nib sizes: 0.13, 0.18, 0.25, 0.35, 0.5, 0.7, 1.0, 1.5, and 2.0 mm. However, the International Organization for Standardization (ISO) called for four pen widths and set a colour code for each: 0.25 (white), 0.35 (yellow), 0.5 (brown), 0.7 (blue); these nibs produced lines that related to various text character heights and the ISO paper sizes.

All ISO paper sizes have the same aspect ratio, one to the square root of 2, meaning that a document designed for any given size can be enlarged or reduced to any other size and will fit perfectly. Given this ease of changing sizes, it is of course common to copy or print a given document on different sizes of paper, especially within a series, e.g. a drawing on A3 may be enlarged to A2 or reduced to A4.

The U.S. customary "A-size" corresponds to "letter" size, and "B-size" corresponds to "ledger" or "tabloid" size. There were also once British paper sizes, which went by names rather than alphanumeric designations.

American Society of Mechanical Engineers (ASME) ANSI/ASME Y14.1, Y14.2, Y14.3, and Y14.5 are commonly referenced standards in the U.S.

Technical lettering

Technical lettering is the process of forming letters, numerals, and other characters in technical drawing. It is used to describe, or provide detailed specifications for, an object. With the goals of legibility and uniformity, styles are standardized and lettering ability has little relationship to normal writing ability. Engineering drawings use a Gothic sans-serif script, formed by a series of short strokes. Lower case letters are rare in most drawings of machines. ISO Lettering templates, designed for use with technical pens and pencils, and to suit ISO paper sizes, produce lettering characters to an international standard. The stroke thickness is related to the character height (for example, 2.5mm high characters would have a stroke thickness - pen nib size - of 0.25mm, 3.5 would use a 0.35mm pen and so forth). The ISO character set (font) has a seriffed one, a barred seven, an open four, six, and nine, and a round topped three, that improves legibility when, for example, an A0 drawing has been reduced to A1 or even A3 (and perhaps enlarged back or reproduced/faxed/ microfilmed &c). When CAD drawings became more popular, especially using US American software, such as AutoCAD, the nearest font to this ISO standard font was Romantic Simplex (RomanS) - a proprietary shx font) with a manually adjusted width factor (over ride) to make it look as near to the ISO lettering for the drawing board. However, with the closed four, and arced six and nine, romans.shx typeface could be difficult to read in reductions. In more recent revisions of software packages, the TrueType font ISOCPEUR reliably reproduces the original drawing board lettering stencil style, however, many drawings have switched to the ubiquitous Arial.ttf.

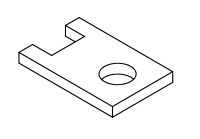
Example of an engineering drawing

Example mechanical drawing

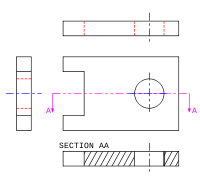
Here is an example of an engineering drawing (an isometric view of the same object is shown above). The different line types are colored for clarity.

• Black = object line and hatching
• Red = hidden line
• Blue = center line of piece or opening
• Magenta = phantom line or cutting plane line

Sectional views are indicated by the direction of arrows, as in the example right side.

Applied Electronic

Semiconductor

For information on semiconductor physics, see semiconductor.

Semiconductor devices are electronic components that exploit the electronic properties of semiconductor materials, principally silicon, germanium, and gallium arsenide, as well as organic semiconductors. Semiconductor devices have replaced thermionic devices (vacuum tubes) in most applications. They use electronic conduction in the solid state as opposed to the gaseous state or thermionic emission in a high vacuum.

Semiconductor devices are manufactured both as single discrete devices and as integrated circuits (ICs), which consist of a number—from a few (as low as two) to billions—of devices manufactured and interconnected on a single semiconductor substrate, or wafer.

Semiconductor materials are useful because their behavior can be easily manipulated by the addition of impurities, known as doping. Semiconductor conductivity can be controlled by introduction of an electric or magnetic field, by exposure to light or heat, or by mechanical deformation of a doped monocrystalline grid; thus, semiconductors can make excellent sensors. Current conduction in a semiconductor occurs via mobile or "free" electrons and holes, collectively known as charge carriers. Doping a semiconductor such as silicon with a small amount of impurity atoms, such as phosphorus or boron, greatly increases the number of free electrons or holes within the semiconductor. When a doped semiconductor contains excess holes it is called "p-type", and when it contains excess free electrons it is known as "n-type", where p (positive for holes) or n (negative for electrons) is the sign of the charge of the majority mobile charge carriers. The semiconductor material used in devices is doped under highly controlled conditions in a fabrication facility, or fab, to control precisely the location and concentration of p- and n-type dopants. The junctions which form where n-type and p-type semiconductors join together are called p–n junctions.

Diode

A semiconductor diode is a device typically made from a single p–n junction. At the junction of a p-type and an n-type semiconductor there forms a depletion region where current conduction is inhibited by the lack of mobile charge carriers. When the device is forward biased (connected with the p-side at higher electric potential than the n-side), this depletion region is diminished, allowing for significant conduction, while only very small current can be achieved when the diode is reverse biased and thus the depletion region expanded.

Exposing a semiconductor to light can generate electron–hole pairs, which increases the number of free carriers and thereby the conductivity. Diodes optimized to take advantage of this phenomenon are known as photodiodes. Compound semiconductor diodes can also be used to generate light, as in light-emitting diodes and laser diodes.

Transistor

Main article: Transistor

An n–p–n bipolar junction transistor structure

Bipolar junction transistors are formed from two p–n junctions, in either n–p–n or p–n–p configuration. The middle, or base, region between the junctions is typically very narrow. The other regions, and their associated terminals, are known as the emitter and the collector. A small current injected through the junction between the base and the emitter changes the properties of the base-collector junction so that it can conduct current even though it is reverse biased. This creates a much larger current between the collector and emitter, controlled by the base-emitter current.

Another type of transistor, the field-effect transistor, operates on the principle that semiconductor conductivity can be increased or decreased by the presence of an electric field. An electric field can increase the number of free electrons and holes in a semiconductor, thereby changing its conductivity. The field may be applied by a reverse-biased p–n junction, forming a junction field-effect transistor (JFET) or by an electrode insulated from the bulk material by an oxide layer, forming a metal–oxide–semiconductor field-effect transistor (MOSFET).

The MOSFET, a solid-state device, is the most used semiconductor device today. The gate electrode is charged to produce an electric field that controls the conductivity of a "channel" between two terminals, called the source and drain. Depending on the type of carrier in the channel, the device may be an n-channel (for electrons) or a p-channel (for holes) MOSFET. Although the MOSFET is named in part for its "metal" gate, in modern devices polysilicon is typically used instead.

Semiconductor device materials

Main article: Semiconductor materials

By far, silicon (Si) is the most widely used material in semiconductor devices. Its combination of low raw material cost, relatively simple processing, and a useful temperature range makes it currently the best compromise among the various competing materials. Silicon used in semiconductor device manufacturing is currently fabricated into boules that are large enough in diameter to allow the production of 300 mm (12 in.) wafers.

Germanium (Ge) was a widely used early semiconductor material but its thermal sensitivity makes it less useful than silicon. Today, germanium is often alloyed with silicon for use in very-high-speed SiGe devices; IBM is a major producer of such devices.

Gallium arsenide (GaAs) is also widely used in high-speed devices but so far, it has been difficult to form large-diameter boules of this material, limiting the wafer diameter to sizes significantly smaller than silicon wafers thus making mass production of GaAs devices significantly more expensive than silicon.

Other less common materials are also in use or under investigation.

Silicon carbide (SiC) has found some application as the raw material for blue light-emitting diodes (LEDs) and is being investigated for use in semiconductor devices that could withstand very high operating temperatures and environments with the presence of significant levels of ionizing radiation. IMPATT diodes have also been fabricated from SiC.

Various indium compounds (indium arsenide, indium antimonide, and indium phosphide) are also being used in LEDs and solid state laser diodes. Selenium sulfide is being studied in the manufacture of photovoltaic solar cells.

The most common use for organic semiconductors is Organic light-emitting diodes.

List of common semiconductor devices

See also: Electronic component § Semiconductors

Two-terminal devices:

• DIAC
• Diode (rectifier diode)
• Gunn diode
• IMPATT diode
• Laser diode
• Light-emitting diode (LED)
• Photocell
• Phototransistor
• PIN diode
• Schottky diode
• Solar cell
• Transient-voltage-suppression diode
• Tunnel diode
• VCSEL
• Zener diode

Three-terminal devices:

• Bipolar transistor
• Darlington transistor
• Field-effect transistor
• Insulated-gate bipolar transistor (IGBT)
• Silicon-controlled rectifier
• Thyristor
• TRIAC
• Unijunction transistor

Four-terminal devices:

• Hall effect sensor (magnetic field sensor)
• Photocoupler (Optocoupler)

Digital Electronic

Digital electronics or digital (electronic) circuits are electronics that handle digital signals- discrete bands of analog levels, rather than by continuous ranges (as used in analogue electronics). All levels within a band of values represent the same numeric value. Because of this discretization, relatively small changes to the analog signal levels due to manufacturing tolerance, signal attenuation or parasitic noise do not leave the discrete envelope, and as a result are ignored by signal state sensing circuitry.

In most cases the number of these states is two, and they are represented by two voltage bands: one near a reference value (typically termed as "ground" or zero volts), and the other a value near the supply voltage. These correspond to the "false" ("0") and "true" ("1") values of the Boolean domain, respectively, yielding binary code.

Digital techniques are useful because it is easier to get an electronic device to switch into one of a number of known states than to accurately reproduce a continuous range of values.

Digital electronic circuits are usually made from large assemblies of logic gates, simple electronic representations of Boolean logic functions.

Electronic Test Equipments

A Tektronix model 475A portable analogue oscilloscope

Electronic test equipment (sometimes called "testgear" or "bench top") is used to create signals and capture responses from electronic Devices Under Test (DUTs). In this way, the proper operation of the DUT can be proven or faults in the device can be traced. Use of electronic test equipment is essential to any serious work on electronics systems.

Practical electronics engineering and assembly requires the use of many different kinds of electronic test equipment ranging from the very simple and inexpensive (such as a test light consisting of just a light bulb and a test lead) to extremely complex and sophisticated such as Automatic Test Equipment. ATE often includes many of these instruments in real and simulated forms.

Generally, more advanced test gear is necessary when developing circuits and systems than is needed when doing production testing or when troubleshooting existing production units in the field.

Types of test equipment

Basic equipment

Agilent commercial digital voltmeter checking a prototype

The following items are used for basic measurement of voltages, currents, and components in the circuit under test.

• Voltmeter (Measures voltage)
• Ohmmeter (Measures resistance)
• Ammeter, e.g. Galvanometer or Milliameter (Measures current)
• Multimeter e.g., VOM (Volt-Ohm-Milliameter) or DMM (Digital Multimeter) (Measures all of the above)

The following are used for stimulus of the circuit under test:

• Power supplies
• Signal generator
• Digital pattern generator
• Pulse generator

Howard piA digital multimeter

The following analyze the response of the circuit under test:

• Oscilloscope (Displays voltage as it changes over time)
• Frequency counter (Measures frequency)

And connecting it all together:

• Test probes

Advanced or less commonly used equipment

Meters

• Solenoid voltmeter (Wiggy)
• Clamp meter (current transducer)
• Wheatstone bridge (Precisely measures resistance)
• Capacitance meter (Measures capacitance)
• LCR meter (Measures inductance, capacitance, resistance and combinations thereof)
• EMF Meter (Measures Electric and Magnetic Fields)
• Electrometer (Measures charge)

Probes

A multimeter with a built in clampfacility. Pushing the large button at the bottom opens the lower jaw of the clamp, allowing the clamp to be placed around a conductor (wire).

• RF probe
• Signal tracer

Analyzers

• Logic analyzer (Tests digital circuits)
• Spectrum analyzer (SA) (Measures spectral energy of signals)
• Protocol analyzer (Tests functionality, performance and conformance of protocols)
• Vector signal analyzer (VSA) (Like the SA but it can also perform many more useful digital demodulation functions)
• Time-domain reflectometer (Tests integrity of long cables)
• Semiconductor curve tracer

Signal-generating devices

Leader Instruments LSG-15 signal generator.

• Signal generator
• Frequency synthesiser
• Function generator
• Digital pattern generator
• Pulse generator
• Signal injector

Miscellaneous devices

• Boxcar averager
• Continuity tester
• Cable tester
• Hipot tester
• Network analyzer (used to characterize an electrical network of components)
• Test light
• Transistor tester
• Tube tester

Applied Electrical

Electrical engineering is a field of engineering that generally deals with the study and application of electricity, electronics, and electromagnetism. This field first became an identifiable occupation in the latter half of the 19th century after commercialization of the electric telegraph, the telephone, and electric power distribution and use. Subsequently, broadcasting and recording media made electronics part of daily life. The invention of the transistor, and later the integrated circuit, brought down the cost of electronics to the point they can be used in almost any household object.

Electrical engineering has now subdivided into a wide range of subfields including electronics, digital computers, power engineering, telecommunications, control systems, radio-frequency engineering, signal processing, instrumentation, and microelectronics. The subject of electronic engineering is often treated as its own subfield but it intersects with all the other subfields, including the power electronics of power engineering.

Electrical engineers typically hold a degree in electrical engineering or electronic engineering. Practicing engineers may have professional certification and be members of a professional body. Such bodies include the Institute of Electrical and Electronics Engineers (IEEE) and the Institution of Engineering and Technology (professional society) (IET).

Electrical engineers work in a very wide range of industries and the skills required are likewise variable. These range from basic circuit theory to the management skills required of a project manager. The tools and equipment that an individual engineer may need are similarly variable, ranging from a simple voltmeter to a top end analyzer to sophisticated design and manufacturing software.

Direct Current (DC)

Direct current (DC) is the unidirectional flow of electric charge. Direct current is produced by sources such as batteries, thermocouples, solar cells, and commutator-type electric machines of the dynamo type. Direct current may flow in a conductor such as a wire, but can also flow through semiconductors, insulators, or even through a vacuum as in electron or ion beams. The electric current flows in a constant direction, distinguishing it from alternating current (AC). A term formerly used for this type of current was galvanic current.

The abbreviations AC and DC are often used to mean simply alternating and direct, as when they modify current or voltage.

Direct current may be obtained from an alternating current supply by use of a current-switching arrangement called a rectifier, which contains electronic elements (usually) or electromechanical elements (historically) that allow current to flow only in one direction. Direct current may be made into alternating current with an inverter or a motor-generator set.

The first commercial electric power transmission (developed by Thomas Edison in the late nineteenth century) used direct current. Because of the significant advantages of alternating current over direct current in transforming and transmission, electric power distribution is nearly all alternating current today. In the mid-1950s, high-voltage direct current transmission was developed, and is now an option instead of long-distance high voltage alternating current systems. For long distance underseas cables (e.g. between countries, such as NorNed), this DC option is the only technically feasible option. For applications requiring direct current, such as third rail power systems, alternating current is distributed to a substation, which utilizes a rectifier to convert the power to direct current. See War of Currents.

Direct current is used to charge batteries, and in nearly all electronic systems, as the power supply. Very large quantities of direct-current power are used in production of aluminum and other electrochemical processes. Direct current is used for some railway propulsion, especially in urban areas. High-voltage direct current is used to transmit large amounts of power from remote generation sites or to interconnect alternating current power grids.

Various definitions

Types of direct current

The term DC is used to refer to power systems that use only one polarity of voltage or current, and to refer to the constant, zero-frequency, or slowly varying local mean value of a voltage or current. For example, the voltage across a DC voltage source is constant as is the current through a DC current source. The DC solution of an electric circuit is the solution where all voltages and currents are constant. It can be shown that any stationary voltage or current waveform can be decomposed into a sum of a DC component and a zero-mean time-varying component; the DC component is defined to be the expected value, or the average value of the voltage or current over all time.

Although DC stands for "direct current", DC often refers to "constant polarity". Under this definition, DC voltages can vary in time, as seen in the raw output of a rectifier or the fluctuating voice signal on a telephone line.

Some forms of DC (such as that produced by a voltage regulator) have almost no variations in voltage, but may still have variations in output power and current.

Circuits

A direct current circuit is an electrical circuit that consists of any combination of constant voltage sources, constant current sources, and resistors. In this case, the circuit voltages and currents are independent of time. A particular circuit voltage or current does not depend on the past value of any circuit voltage or current. This implies that the system of equations that represent a DC circuit do not involve integrals or derivatives with respect to time.

If a capacitor or inductor is added to a DC circuit, the resulting circuit is not, strictly speaking, a DC circuit. However, most such circuits have a DC solution. This solution gives the circuit voltages and currents when the circuit is in DC steady state. Such a circuit is represented by a system of differential equations. The solution to these equations usually contain a time varying or transient part as well as constant or steady state part. It is this steady state part that is the DC solution. There are some circuits that do not have a DC solution. Two simple examples are a constant current source connected to a capacitor and a constant voltage source connected to an inductor.

In electronics, it is common to refer to a circuit that is powered by a DC voltage source such as a battery or the output of a DC power supply as a DC circuit even though what is meant is that the circuit is DC powered.

Applications

Direct-current installations usually have different types of sockets, connectors, switches, and fixtures, mostly due to the low voltages used, from those suitable for alternating current. It is usually important with a direct-current appliance not to reverse polarity unless the device has a diode bridge to correct for this (most battery-powered devices do not).

This symbol is found on many electronic devices that either require or produce direct current.

The Unicode code point for the direct current symbol, found in the Miscellaneous Technical block, is U+2393 (⎓).

DC is commonly found in many extra-low voltage applications and some low-voltage applications, especially where these are powered by batteries, which can produce only DC, or solar power systems, since solar cells can produce only DC. Most automotive applications use DC, although the alternator is an AC device which uses a rectifier to produce DC. Most electronic circuits require a DC power supply. Applications using fuel cells (mixing hydrogen and oxygen together with a catalyst to produce electricity and water as byproducts) also produce only DC.

The vast majority of automotive applications use "12-volt" DC power; a few have a 6 V or a 42 V electrical system.

Light aircraft electrical systems are typically 12 V or 28 V.

Through the use of a DC-DC converter, high DC voltages such as 48 V to 72 V DC can be stepped down to 36 V, 24 V, 18 V, 12 V or 5 V to supply different loads. In a telecommunications system operating at 48 V DC, it is generally more efficient to step voltage down to 12 V to 24 V DC with a DC-DC converter and power equipment loads directly at their native DC input voltages versus operating a 48 V DC to 120 V AC inverter to provide power to equipment.

Many telephones connect to a twisted pair of wires, and use a bias tee to internally separate the AC component of the voltage between the two wires (the audio signal) from the DC component of the voltage between the two wires (used to power the phone).

Telephone exchange communication equipment, such as DSLAM, uses standard −48 V DC power supply. The negative polarity is achieved by grounding the positive terminal of power supply system and the battery bank. This is done to prevent electrolysis depositions.

See also

• Electric current
• High-voltage direct current power transmission.
• Alternating current
• DC offset
• Neutral direct-current telegraph system

Alternating Current

Alternating current (AC), is an electric current in which the flow of electric charge periodically reverses direction, whereas in direct current (DC, also dc), the flow of electric charge is only in one direction. The abbreviations AC and DC are often used to mean simply alternating and direct, as when they modify current or voltage.

AC is the form in which electric power is delivered to businesses and residences. The usual waveform of alternating current in most electric power circuits is a sine wave. In certain applications, different waveforms are used, such as triangular or square waves. Audio and radio signals carried on electrical wires are also examples of alternating current. These types of alternating current carry information encoded (or modulated) onto the AC signal, such as sound (audio) or images (video).

Transmission, distribution, and domestic power supply

Main articles: Electric power transmission and Electricity distribution

The reason that electric power is distributed as alternating current is that AC voltage may be increased or decreased with a transformer. This allows the power to be transmitted through power lines efficiently at high voltage, which reduces the power lost as heat due to resistance of the wire, and the voltage to be reduced to safe levels for use by the customer. Use of a higher voltage leads to significantly more efficient transmission of power. The power losses ($P_{\rm {L}}$) in a conductor are a product of the square of the current (I) and the resistance (R) of the conductor, described by the formula

$P_{\rm {L}}=I^{2}R\,.$

This means that when transmitting a fixed power on a given wire, if the current is doubled, the power loss will be four times greater.

The power transmitted is equal to the product of the current and the voltage (assuming no phase difference); that is,

$P_{\rm {T}}=IV\,.$

Thus, the same amount of power can be transmitted with a lower current by increasing the voltage. It is therefore advantageous when transmitting large amounts of power to distribute the power with high voltages (often hundreds of kilovolts).

High voltage transmission lines deliver power from electric generation plants over long distances using alternating current. These lines are located in eastern Utah.

However, high voltages also have disadvantages, the main one being the increased insulation required, and generally increased difficulty in their safe handling. In a power plant, power is generated at a convenient voltage for the design of a generator, and then stepped up to a high voltage for transmission. Near the loads, the transmission voltage is stepped down to the voltages used by equipment. Consumer voltages vary depending on the country and size of load, but generally motors and lighting are built to use up to a few hundred volts between phases.

The utilization voltage delivered to equipment such as lighting and motor loads is standardized, with an allowable range of voltage over which equipment is expected to operate. Standard power utilization voltages and percentage tolerance vary in the different mains power systems found in the world.

Modern high-voltage direct-current (HVDC) electric power transmission systems contrast with the more common alternating-current systems as a means for the efficient bulk transmission of electrical power over long distances. HVDC systems, however, tend to be more expensive and less efficient over shorter distances than transformers. Transmission with high voltage direct current was not feasible when Edison, Westinghouse and Tesla were designing their power systems, since there was then no way to economically convert AC power to DC and back again at the necessary voltages.

Three-phase electrical generation is very common. The simplest case is three separate coils in the generator stator that are physically offset by an angle of 120° to each other. Three current waveforms are produced that are equal in magnitude and 120° out of phase to each other. If coils are added opposite to these (60° spacing), they generate the same phases with reverse polarity and so can be simply wired together.

In practice, higher "pole orders" are commonly used. For example, a 12-pole machine would have 36 coils (10° spacing). The advantage is that lower speeds can be used. For example, a 2-pole machine running at 3600 rpm and a 12-pole machine running at 600 rpm produce the same frequency. This is much more practical for larger machines.

If the load on a three-phase system is balanced equally among the phases, no current flows through the neutral point. Even in the worst-case unbalanced (linear) load, the neutral current will not exceed the highest of the phase currents. Non-linear loads (e.g., computers) may require an oversized neutral bus and neutral conductor in the upstream distribution panel to handle harmonics. Harmonics can cause neutral conductor current levels to exceed that of one or all phase conductors.

For three-phase at utilization voltages a four-wire system is often used. When stepping down three-phase, a transformer with a Delta (3-wire) primary and a Star (4-wire, center-earthed) secondary is often used so there is no need for a neutral on the supply side.

For smaller customers (just how small varies by country and age of the installation) only a single phase and the neutral or two phases and the neutral are taken to the property. For larger installations all three phases and the neutral are taken to the main distribution panel. From the three-phase main panel, both single and three-phase circuits may lead off.

Three-wire single-phase systems, with a single center-tapped transformer giving two live conductors, is a common distribution scheme for residential and small commercial buildings in North America. This arrangement is sometimes incorrectly referred to as "two phase". A similar method is used for a different reason on construction sites in the UK. Small power tools and lighting are supposed to be supplied by a local center-tapped transformer with a voltage of 55 V between each power conductor and earth. This significantly reduces the risk of electric shock in the event that one of the live conductors becomes exposed through an equipment fault whilst still allowing a reasonable voltage of 110 V between the two conductors for running the tools.

A third wire, called the bond (or earth) wire, is often connected between non-current-carrying metal enclosures and earth ground. This conductor provides protection from electric shock due to accidental contact of circuit conductors with the metal chassis of portable appliances and tools. Bonding all non-current-carrying metal parts into one complete system ensures there is always a low electrical impedance path to ground sufficient to carry any fault current for as long as it takes for the system to clear the fault. This low impedance path allows the maximum amount of fault current, causing the overcurrent protection device (breakers, fuses) to trip or burn out as quickly as possible, bringing the electrical system to a safe state. All bond wires are bonded to ground at the main service panel, as is the Neutral/Identified conductor if present.

AC power supply frequencies

Further information: Mains power around the world

The frequency of the electrical system varies by country and sometimes within a country; most electric power is generated at either 50 or 60 hertz. Some countries have a mixture of 50 Hz and 60 Hz supplies, notably electricity power transmission in Japan.

A low frequency eases the design of electric motors, particularly for hoisting, crushing and rolling applications, and commutator-type traction motors for applications such as railways. However, low frequency also causes noticeable flicker in arc lamps and incandescent light bulbs. The use of lower frequencies also provided the advantage of lower impedance losses, which are proportional to frequency. The original Niagara Falls generators were built to produce 25 Hz power, as a compromise between low frequency for traction and heavy induction motors, while still allowing incandescent lighting to operate (although with noticeable flicker). Most of the 25 Hz residential and commercial customers for Niagara Falls power were converted to 60 Hz by the late 1950s, although some 25 Hz industrial customers still existed as of the start of the 21st century. 16.7 Hz power (formerly 16 2/3 Hz) is still used in some European rail systems, such as in Austria, Germany, Norway, Sweden and Switzerland.

Off-shore, military, textile industry, marine, aircraft, and spacecraft applications sometimes use 400 Hz, for benefits of reduced weight of apparatus or higher motor speeds.

Computer mainframe systems are often powered by 415 Hz, using customer-supplied 35 or 70 KVA motor-generator sets. Smaller mainframes may have an internal 415 Hz M-G set. In any case, the input to the M-G set is the local customary voltage and frequency, variously 200 (Japan), 208, 240 (North America), 380, 400 or 415 (Europe) volts, and variously 50 or 60 Hz.

Effects at high frequencies

Main article: Skin effect

A direct current flows uniformly throughout the cross-section of a uniform wire. An alternating current of any frequency is forced away from the wire's center, toward its outer surface. This is because the acceleration of an electric charge in an alternating current produces waves of electromagnetic radiation that cancel the propagation of electricity toward the center of materials with high conductivity. This phenomenon is called skin effect.

At very high frequencies the current no longer flows in the wire, but effectively flows on the surface of the wire, within a thickness of a few skin depths. The skin depth is the thickness at which the current density is reduced by 63%. Even at relatively low frequencies used for power transmission (50–60 Hz), non-uniform distribution of current still occurs in sufficiently thick conductors. For example, the skin depth of a copper conductor is approximately 8.57 mm at 60 Hz, so high current conductors are usually hollow to reduce their mass and cost.

Since the current tends to flow in the periphery of conductors, the effective cross-section of the conductor is reduced. This increases the effective AC resistance of the conductor, since resistance is inversely proportional to the cross-sectional area. The AC resistance often is many times higher than the DC resistance, causing a much higher energy loss due to ohmic heating (also called I²R loss).

Techniques for reducing AC resistance

For low to medium frequencies, conductors can be divided into stranded wires, each insulated from one other, and the relative positions of individual strands specially arranged within the conductor bundle. Wire constructed using this technique is called Litz wire. This measure helps to partially mitigate skin effect by forcing more equal current throughout the total cross section of the stranded conductors. Litz wire is used for making high-Q inductors, reducing losses in flexible conductors carrying very high currents at lower frequencies, and in the windings of devices carrying higher radio frequency current (up to hundreds of kilohertz), such as switch-mode power supplies and radio frequency transformers.

Techniques for reducing radiation loss

As written above, an alternating current is made of electric charge under periodic acceleration, which causes radiation of electromagnetic waves. Energy that is radiated is lost. Depending on the frequency, different techniques are used to minimize the loss due to radiation.

Twisted pairs

At frequencies up to about 1 GHz, pairs of wires are twisted together in a cable, forming a twisted pair. This reduces losses from electromagnetic radiation and inductive coupling. A twisted pair must be used with a balanced signalling system, so that the two wires carry equal but opposite currents. Each wire in a twisted pair radiates a signal, but it is effectively cancelled by radiation from the other wire, resulting in almost no radiation loss.

Coaxial cables

Coaxial cables are commonly used at audio frequencies and above for convenience. A coaxial cable has a conductive wire inside a conductive tube, separated by a dielectric layer. The current flowing on the inner conductor is equal and opposite to the current flowing on the inner surface of the tube. The electromagnetic field is thus completely contained within the tube, and (ideally) no energy is lost to radiation or coupling outside the tube. Coaxial cables have acceptably small losses for frequencies up to about 5 GHz. For microwave frequencies greater than 5 GHz, the losses (due mainly to the electrical resistance of the central conductor) become too large, making waveguides a more efficient medium for transmitting energy. Coaxial cables with an air rather than solid dielectric are preferred as they transmit power with lower loss.

Waveguides

Waveguides are similar to coax cables, as both consist of tubes, with the biggest difference being that the waveguide has no inner conductor. Waveguides can have any arbitrary cross section, but rectangular cross sections are the most common. Because waveguides do not have an inner conductor to carry a return current, waveguides cannot deliver energy by means of an electric current, but rather by means of a guided electromagnetic field. Although surface currents do flow on the inner walls of the waveguides, those surface currents do not carry power. Power is carried by the guided electromagnetic fields. The surface currents are set up by the guided electromagnetic fields and have the effect of keeping the fields inside the waveguide and preventing leakage of the fields to the space outside the waveguide.

Waveguides have dimensions comparable to the wavelength of the alternating current to be transmitted, so they are only feasible at microwave frequencies. In addition to this mechanical feasibility, electrical resistance of the non-ideal metals forming the walls of the waveguide cause dissipation of power (surface currents flowing on lossy conductors dissipate power). At higher frequencies, the power lost to this dissipation becomes unacceptably large.

Fiber optics

At frequencies greater than 200 GHz, waveguide dimensions become impractically small, and the ohmic losses in the waveguide walls become large. Instead, fiber optics, which are a form of dielectric waveguides, can be used. For such frequencies, the concepts of voltages and currents are no longer used.

Mathematics of AC voltages

A sine wave, over one cycle (360°). The dashed line represents the root mean square (RMS) value at about 0.707

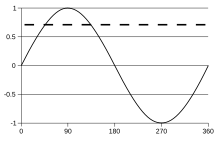
Alternating currents are accompanied (or caused) by alternating voltages. An AC voltage v can be described mathematically as a function of time by the following equation:

$v(t)=V_{\mathrm {peak} }\cdot \sin(\omega t)$,

where

• $\displaystyle V_{\rm {peak}}$ is the peak voltage (unit: volt),
• $\displaystyle \omega$ is the angular frequency (unit: radians per second)
  • The angular frequency is related to the physical frequency, $\displaystyle f$ (unit = hertz), which represents the number of cycles per second, by the equation $\displaystyle \omega =2\pi f$.
• $\displaystyle t$ is the time (unit: second).

The peak-to-peak value of an AC voltage is defined as the difference between its positive peak and its negative peak. Since the maximum value of $\sin(x)$ is +1 and the minimum value is −1, an AC voltage swings between $+V_{\rm {peak}}$ and $-V_{\rm {peak}}$. The peak-to-peak voltage, usually written as $V_{\rm {pp}}$ or $V_{\rm {P-P}}$, is therefore $V_{\rm {peak}}-(-V_{\rm {peak}})=2V_{\rm {peak}}$.

Power

Main article: AC power

The relationship between voltage and the power delivered is

$p(t)={\frac {v^{2}(t)}{R}}$ where $R$ represents a load resistance.

Rather than using instantaneous power, $p(t)$, it is more practical to use a time averaged power (where the averaging is performed over any integer number of cycles). Therefore, AC voltage is often expressed as a root mean square (RMS) value, written as $V_{\rm {rms}}$, because

$P_{\rm {time~averaged}}={\frac {{V^{2}}_{\rm {rms}}}{R}}.$

Power oscillation

$v(t)=V_{\mathrm {peak} }\sin(\omega t)$

$i(t)={\frac {v(t)}{R}}={\frac {V_{\mathrm {peak} }}{R}}\sin(\omega t)$

$P(t)=v(t)\ i(t)={\frac {(V_{\mathrm {peak} })^{2}}{R}}\sin ^{2}(\omega t)$

By the following trigonometric identity, the power oscillation is double frequency of the voltage.

$\sin ^{2}x={\frac {1-\cos 2x}{2}}$

Root mean square

For a sinusoidal voltage:

${\begin{aligned}V_{\mathrm {rms} }&={\sqrt {{\frac {1}{T}}\int _{0}^{T}[{V_{pk}\sin(\omega t+\phi )]^{2}dt}}}\\&=V_{pk}{\sqrt {{\frac {1}{2T}}\int _{0}^{T}[{1-\cos(2\omega t+2\phi )]dt}}}\\&=V_{pk}{\sqrt {{\frac {1}{2T}}\int _{0}^{T}{dt}}}\\&={\frac {V_{pk}}{\sqrt {2}}}\end{aligned}}$

The factor ${\sqrt {2}}$ is called the crest factor, which varies for different waveforms.

• For a triangle waveform centered about zero

$V_{\mathrm {rms} }={\frac {V_{\mathrm {peak} }}{\sqrt {3}}}.$

• For a square waveform centered about zero

$\displaystyle V_{\mathrm {rms} }=V_{\mathrm {peak} }.$

• For an arbitrary periodic waveform $v(t)$ of period $T$:

$V_{\mathrm {rms} }={\sqrt {{\frac {1}{T}}\int _{0}^{T}{[v(t)]^{2}dt}}}.$

Example

To illustrate these concepts, consider a 230 V AC mains supply used in many countries around the world. It is so called because its root mean square value is 230 V. This means that the time-averaged power delivered is equivalent to the power delivered by a DC voltage of 230 V. To determine the peak voltage (amplitude), we can rearrange the above equation to:

$V_{\mathrm {peak} }={\sqrt {2}}\ V_{\mathrm {rms} }.$

For 230 V AC, the peak voltage $\scriptstyle V_{\mathrm {peak} }$ is therefore $\scriptstyle 230V\times {\sqrt {2}}$, which is about 325 V.

Transformers

Alternating current systems can use transformers to change voltage from low to high level and back, allowing generation and consumption at low voltages but transmission, possibly over great distances, at high voltage, with savings in the cost of conductors and energy losses.

A bipolar open-core power transformer developed by Lucien Gaulard and John Dixon Gibbs was demonstrated in London in 1881, and attracted the interest of Westinghouse. They also exhibited the invention in Turin in 1884. However these early induction coils with open magnetic circuits are inefficient at transferring power to loads. Until about 1880, the paradigm for AC power transmission from a high voltage supply to a low voltage load was a series circuit. Open-core transformers with a ratio near 1:1 were connected with their primaries in series to allow use of a high voltage for transmission while presenting a low voltage to the lamps. The inherent flaw in this method was that turning off a single lamp (or other electric device) affected the voltage supplied to all others on the same circuit. Many adjustable transformer designs were introduced to compensate for this problematic characteristic of the series circuit, including those employing methods of adjusting the core or bypassing the magnetic flux around part of a coil.
The direct current systems did not have these drawbacks, giving it significant advantages over early AC systems.

Pioneers

The prototype of ZBD. transformer is on display at the Széchenyi István Memorial Exhibition, Nagycenk, Hungary

The Hungarian "ZBD" Team (Károly Zipernowsky, Ottó Bláthy, Miksa Déri). They were the inventors of the first high efficiency, closed core shunt connection transformer. The three also invented the modern power distribution system: Instead of former series connection they connect transformers that supply the appliances in parallel to the main line.Blathy invented the AC Wattmeter, and they invented the essential Constant Voltage Generator.

In the autumn of 1884, Károly Zipernowsky, Ottó Bláthy and Miksa Déri (ZBD), three engineers associated with the Ganz factory, determined that open-core devices were impractical, as they were incapable of reliably regulating voltage. In their joint 1885 patent applications for novel transformers (later called ZBD transformers), they described two designs with closed magnetic circuits where copper windings were either a) wound around iron wire ring core or b) surrounded by iron wire core. In both designs, the magnetic flux linking the primary and secondary windings traveled almost entirely within the confines of the iron core, with no intentional path through air (see Toroidal cores below). The new transformers were 3.4 times more efficient than the open-core bipolar devices of Gaulard and Gibbs.

The Ganz factory in 1884 shipped the world's first five high-efficiency AC transformers. This first unit had been manufactured to the following specifications: 1,400 W, 40 Hz, 120:72 V, 11.6:19.4 A, ratio 1.67:1, one-phase, shell form.

The ZBD patents included two other major interrelated innovations: one concerning the use of parallel connected, instead of series connected, utilization loads, the other concerning the ability to have high turns ratio transformers such that the supply network voltage could be much higher (initially 1,400 to 2,000 V) than the voltage of utilization loads (100 V initially preferred). When employed in parallel connected electric distribution systems, closed-core transformers finally made it technically and economically feasible to provide electric power for lighting in homes, businesses and public spaces.

The other essential milestone was the introduction of 'voltage source, voltage intensive' (VSVI) systems' by the invention of constant voltage generators in 1885. Ottó Bláthy also invented the first AC electricity meter.

The AC power systems was developed and adopted rapidly after 1886 due to its ability to distribute electricity efficiently over long distances, overcoming the limitations of the direct current system. In 1886, the ZBD engineers designed, and the Ganz factory supplied electrical equipment for, the world's first power station that used AC generators to power a parallel connected common electrical network, the steam-powered Rome-Cerchi power plant. The reliability of the AC technology received impetus after the Ganz Works electrified a large European metropolis: Rome in 1886.

The city lights of Prince George, British Columbia viewed in a motion blurred exposure. The AC blinking causes the lines to be dotted rather than continuous.

Westinghouse Early AC System 1887
(US patent 373035)

In the UK Sebastian de Ferranti, who had been developing AC generators and transformers in London since 1882, redesigned the AC system at the Grosvenor Gallery power station in 1886 for the London Electric Supply Corporation (LESCo) including alternators of his own design and transformer designs similar to Gaulard and Gibbs. In 1890 he designed their power station at Deptford and converted the Grosvenor Gallery station across the Thames into an electrical substation, showing the way to integrate older plants into a universal AC supply system.

In the US William Stanley, Jr. designed one of the first practical devices to transfer AC power efficiently between isolated circuits. Using pairs of coils wound on a common iron core, his design, called an induction coil, was an early (1885) transformer. Stanley also worked on engineering and adapting European designs such as the Gaulard and Gibbs transformer for US entrepreneur George Westinghouse who started building AC systems in 1886. The spread of Westinghouse and other AC systems triggered a push back in late 1887 by Thomas Edison (a proponent of direct current) who attempted to discredit alternating current as too dangerous in a public campaign called the "War of Currents".

In 1888 alternating current systems gained further viability with introduction of a functional AC motor, something these systems had lacked up till then. The design, an induction motor, was independently invented by Galileo Ferraris and Nikola Tesla (with Tesla's design being licensed by Westinghouse in the US). This design was further developed into the modern practical three-phase form by Mikhail Dolivo-Dobrovolsky and Charles Eugene Lancelot Brown.

The Ames Hydroelectric Generating Plant (spring of 1891) and the original Niagara Falls Adams Power Plant (August 25, 1895) were among the first hydroelectric AC-power plants. The first commercial power plant in the United States using three-phase alternating current was the hydroelectric Mill Creek No. 1 Hydroelectric Plant near Redlands, California, in 1893 designed by Almirian Decker. Decker's design incorporated 10,000-volt three-phase transmission and established the standards for the complete system of generation, transmission and motors used today.

The Jaruga Hydroelectric Power Plant in Croatia was set in operation on 28 August 1895. The two generators (42 Hz, 550 kW each) and the transformers were produced and installed by the Hungarian company Ganz. The transmission line from the power plant to the City of Šibenik was 11.5 kilometers (7.1 mi) long on wooden towers, and the municipal distribution grid 3000 V/110 V included six transforming stations.

Alternating current circuit theory developed rapidly in the latter part of the 19th and early 20th century. Notable contributors to the theoretical basis of alternating current calculations include Charles Steinmetz, Oliver Heaviside, and many others. Calculations in unbalanced three-phase systems were simplified by the symmetrical components methods discussed by Charles Legeyt Fortescue in 1918.

See also

• AC power
• Direct current
• Electric current
• Electrical wiring
• Heavy-duty power plugs
• Hertz
• Mains power systems
• AC power plugs and sockets
• Utility frequency
• War of Currents
• AC/DC receiver design